ARCHIVES
- Title
- Tensor tree learns hidden relational structures in data to construct generative models
- Author
- Kenji Harada, Tsuyoshi Okubo, Naoki Kawashima
- Abstract
- Based on the tensor tree network with the Born machine framework, we propose a general method for constructing a generative model by expressing the target distribution function as the amplitude of the quantum wave function represented by a tensor tree. The key idea is dynamically optimizing the tree structure that minimizes the bond mutual information. The proposed method offers enhanced performance and uncovers hidden relational structures in the target data. We illustrate potential practical applications with four examples: (i) random patterns, (ii) QMNIST handwritten digits, (iii) Bayesian networks, and (iv) the pattern of stock price fluctuation pattern in S\&P500. In (i) and (ii), the strongly correlated variables were concentrated near the center of the network; in (iii), the causality pattern was identified; and in (iv), a structure corresponding to the eleven sectors emerged.
- Comments
- 10 pages, 3 figures
- Citation
- Kenji Harada, Tsuyoshi Okubo, and Naoki Kawashima, Machine Learning: Science and Technology 6 025002(2025)
- DOI
- 10.1088/2632-2153/adc2c7
- Code
- Adaptive Tensor Tree Generative Modeling
- Date
- December 13th, 2024
- Seminar
- Theory seminar at ISSP, Univ. of Tokyo
- Title
- Tensor tree learns hidden relational structure in data to construct generative models
- Abstract
-
Generative modeling is a significant machine learning technique that constructs the probability distribution of a dataset, owing to its wide range of applications across various problems. Recently, there has been extensive research into generative modeling on quantum computers, referred to as Born machines. This approach utilizes the output of projective measurement of quantum states for stochastic samplings.
We propose a general method for constructing a generative model based on the tree tensor network within the Born machine framework. The core idea is to optimize the tree structure dynamically to minimize the bond mutual information. We demonstrate potential applications with four examples: (1) Random bit sequences with long-range correlation, (2) Images of handwritten digits from the QMNIST dataset, (3) Bayesian networks, (4) Stock price fluctuations in the S&P 500.
Our method significantly enhances performance and reveals hidden relational structures in the target data, paving the way for future improvements and advancements.
- Reference
- arXiv:2408.10669
- Code
- Adaptive Tensor Tree Generative Modeling
- Title
- Tensor tree learns hidden relational structures in data to construct generative models
- Author
- Kenji Harada, Tsuyoshi Okubo, Naoki Kawashima
- Abstract
- Based on the tensor tree network with the Born machine framework, we propose a general method for constructing a generative model by expressing the target distribution function as the quantum wave function amplitude represented by a tensor tree. The key idea is dynamically optimizing the tree structure that minimizes the bond mutual information. The proposed method offers enhanced performance and uncovers hidden relational structures in the target data. We illustrate potential practical applications with four examples: (i) random patterns, (ii) QMNIST hand-written digits, (iii) Bayesian networks, and (iv) the stock price fluctuation pattern in S&P500. In (i) and (ii), strongly correlated variables were concentrated near the center of the network; in (iii), the causality pattern was identified; and, in (iv), a structure corresponding to the eleven sectors emerged.
- Comments
- 9 pages, 3 figures
- Preprint
- arXiv:2408.10669
- Code
- Adaptive Tensor Tree Generative Modeling
- Dates
- March 25-29, 2024
- Conference
- SQAI-NCTS Workshop on Tensor Network and Quantum Embedding (Hongo Campus, The University of Tokyo)
- Title
- Optimizing the structure of tree tensor network for quantum generative modeling using mutual information-based approach
- Abstract
- Generative modeling is a crucial task in the field of machine learning. Recently, there have been several proposals for generative models on quantum devices. We can efficiently optimize generative models defined by tensor network states, but their performance largely depends on the geometrical structure of the tensor network. To tackle this issue, we have proposed an optimization method for the network structure in the tree tensor network class, based on the least mutual information principle. Generative modeling with an optimized network structure has better performance than a fixed network structure. Moreover, by embedding data dependencies into the tree structure based on the least mutual information principle, we can geometrically represent the correlations in the data.
- Book title
- Advanced Mathematical Science for Mobility Society
- Editors
- Kazushi Ikeda, Yoshiumi Kawamura, Kazuhisa Makino, Satoshi Tsujimoto, Nobuo Yamashita, Shintaro Yoshizawa, Hanna Sumita
- Publisher
- Springer Singapore
- Reference
- ISBN 978-981-99-9771-8 ISBN 978-981-99-9772-5 (eBook)
- Title
- Chapter 5 "Application of Tensor Network Formalism for Processing Tensor Data"
- Authors
- Kenji Harada, Hiroaki Matsueda, and Tsuyoshi Okubo
- Web page
- Open access
- Date
- Jan 26, 2024
- Conference
- 2024 Annual Meeting of the Physical Society of Taiwan, Topical Symposia:Many-body systems and advanced numerical methods
- Title
- Optimizing tensor network structure
- Date
- Jan 22, 2024
- Conference
- Mini-workshop: Tensor Network algorithms and applications 2024 (Taipei, Taiwan)
- Title
- Optimizing tensor network structure
- Date
- Aug 22, 2023
- Conference
- Tensor Network States: Algorithms and Applications 2023 (Shanghai, China)
- Title
- Tensor network study of one-dimensional stochastic processes
- Date
- Aug 8, 2023
- Conference
- The 28th International Conference on Statistical Physics, Statphys28 (Tokyot, Japan)
- Title
- Renormalization of non-equilibrium critical points in one-dimensional stochastic processes by tensor networks
- Abstract
-
Non-equilibrium critical points often hold scaling invariance in the spatial and temporal directions. As seen in equilibrium cases, we know various universality classes of non-equilibrium critical points in stochastic processes. However, the direct renormalization of statistical processes is technically difficult. Recently, renormalization using tensor network representation was proposed and extended[1-4], and it is quite successful in equilibrium critical points. We extend the approach to stochastic processes using oblique projectors in the tensor renormalization group with higher-order singular value decomposition[5]. We report the universal property of time-evolution operators of one-dimensional contact processes of which critical points belong to the (1+1)-dimensional directed percolation(DP) universality class. The renormalized time-evolution operator has a universal spectrum structure of the (1+1)-dimensional DP universality class in spatial and temporal directions.
[1] M. Levin and C. P. Nave, Tensor Renormalization Group Approach to Two-Dimensional Classical Lattice Models, Physical Review Letters 99, 120601 (2007).
[2] Z. Y. Xie, J. Chen, M. P. Qin, J. W. Zhu, Y. P. L., and T. Xiang, Coarse-Graining Renormalization by Higher-Order Singular Value Decomposition, Physical Review B 86, 045139 (2012).
[3] G. Evenbly and G. Vidal, Tensor Network Renormalization, Physical Review Letters 115, 180405 (2015).
[4] K. Harada, Entanglement Branching Operator, Physical Review B 97, 045124 (2018).
[5] K. Harada, Universal spectrum structure at nonequilibrium critical points in the (1+1)-dimensional directed percolation, arXiv:2008.10807.
- Title
- Neural network approach to scaling analysis of critical phenomena
- Reference
- Physical Review E 107, 044128 (2023)
- DOI
- 10.1103/PhysRevE.107.044128
- Author
- 10.1103/PhysRevE.107.044128
- Ryosuke Yoneda and Kenji Harada
- Abstract
- Determining the universality class of a system exhibiting critical phenomena is one of the central problems in physics. There are several methods to determine this universality class from data. As methods to collapse plots onto scaling functions, polynomial regression, which is less accurate, and Gaussian process regression, which provides high accuracy and flexibility but is computationally expensive, have been proposed. In this paper, we propose a regression method using a neural network. The computational complexity is linear only in the number of data points. We demonstrate the proposed method for the finite-size scaling analysis of critical phenomena in the two-dimensional Ising model and bond percolation problem to confirm the performance. This method efficiently obtains the critical values with accuracy in both cases.
- Comments
- 10 pages, 10 figures
- Preprint
- arXiv.2209.01777
- Title
- Quantum critical dynamics in the two-dimensional transverse Ising model
- Reference
- Physical Review Research 5, 013186 (2023)
- DOI
- 10.1103/PhysRevResearch.5.013186
- Author
- 10.1103/PhysRevResearch.5.013186
- Chisa Hotta, Tempei Yoshida, and Kenji Harada
- Abstract
- In the vicinity of the quantum critical point (QCP), thermodynamic properties diverge toward zero temperature governed by universal exponents. Although this fact is well known, how it is reflected in quantum dynamics has not been addressed. The QCP of the transverse Ising model on a triangular lattice is an ideal platform to test the issue, since it has an experimental realization, the dielectrics being realized in an organic dimer Mott insulator, κ−ET2X, where a quantum electric dipole represents the Ising degrees of freedom. We track the Glauber-type dynamics of the model by constructing a kinetic protocol based on the quantum Monte Carlo method. The dynamical susceptibility takes the form of the Debye function and shows a significant peak narrowing in approaching a QCP due to the divergence of the relaxation timescale. It explains the anomaly of dielectric constants observed in the organic materials, indicating that the material is very near the ferroelectric QCP. We disclose how the dynamical and other critical exponents develop near QCP beyond the simple field theory.
- Comments
- 12 pages, 8 figures
- Preprint
- arXiv:2209.11599
- Title
- Quantum Circuit Simulation by SGEMM Emulation on Tensor Cores and Automatic Precision Selection
- Reference
- ISC 2023
- Author
- Hiryuki Ootomo, Hidetaka Manabe, Kenji Harada, and Rio Yokota
- Abstract
- Quantum circuit simulation provides the foundation for the development of quantum algorithms and the verification of quantum supremacy. Among the various methods for quantum circuit simulation, tensor network contraction has been increasing in popularity due to its ability to simulate a larger number of qubits. During tensor contraction, the input tensors are reshaped to matrices and computed by a GEMM operation, where these GEMM operations could reach up to 90\% of the total calculation time. GEMM throughput can be improved by utilizing mixed-precision hardware such as Tensor Cores, but straightforward implementation results in insufficient fidelity for deep and large quantum circuits. Prior work has demonstrated that compensated summation with special care of the rounding mode can fully recover the FP32 precision of SGEMM even when using TF32 or FP16 Tensor Cores. The exponent range is a critical issue when applying such techniques to quantum circuit simulation. While TF32 supports almost the same exponent range as FP32, FP16 supports a much smaller exponent range. In this work, we use the exponent range statistics of input tensor elements to select which Tensor Cores we use for the GEMM. We evaluate our method on Random Circuit Sampling (RCS), including Sycamore's quantum circuit, and show that the throughput is 1.86 times higher at maximum while maintaining accuracy.
- Preprint
- arXiv:2303.08989 [quant-ph]
- Title
- Automatic structural optimization of tree tensor networks
- Reference
- Physical Review Research 5, 013031 (2023)
- DOI
- 10.1103/PhysRevResearch.5.013031
- Author
- 10.1103/PhysRevResearch.5.013031
- Toshiya Hikihara, Hiroshi Ueda, Kouichi Okunishi, Kenji Harada, and Tomotoshi Nishino
- Abstract
- Tree tensor network (TTN) provides an essential theoretical framework for the practical simulation of quantum many-body systems, where the network structure defined by the connectivity of the isometry tensors plays a crucial role in improving its approximation accuracy. In this paper, we propose a TTN algorithm that enables us to automatically optimize the network structure by local reconnections of isometries to suppress the bipartite entanglement entropy on their legs. The algorithm can be seamlessly implemented to such a conventional TTN approach as density-matrix renormalization group. We apply the algorithm to the inhomogeneous antiferromagnetic Heisenberg spin chain having a hierarchical spatial distribution of the interactions. We then demonstrate that the entanglement structure embedded in the ground-state of the system can be efficiently visualized as a perfect binary tree in the optimized TTN. Possible improvements and applications of the algorithm are also discussed.
- Comments
- 11 pages, 10 figures, 2 tables
- Preprint
- arXiv:2209.03196
- Title
- Automatic structural optimization of tree tensor networks
- Author
- Toshiya Hikihara, Hiroshi Ueda, Kouichi Okunishi, Kenji Harada, and Tomotoshi Nishino
- Abstract
- Tree tensor network (TTN) provides an essential theoretical framework for the practical simulation of quantum many-body systems, where the network structure defined by the connectivity of the isometry tensors plays a crucial role in improving its approximation accuracy. In this paper, we propose a TTN algorithm that enables us to automatically optimize the network structure by local reconnections of isometries to suppress the bipartite entanglement entropy on their legs. The algorithm can be seamlessly implemented to such a conventional TTN approach as density-matrix renormalization group. We apply the algorithm to the inhomogeneous antiferromagnetic Heisenberg spin chain having a hierarchical spatial distribution of the interactions. We then demonstrate that the entanglement structure embedded in the ground-state of the system can be efficiently visualized as a perfect binary tree in the optimized TTN. Possible improvements and applications of the algorithm are also discussed.
- Comments
- 11 pages, 10 figures, 2 tables
- Preprint
- arXiv:2209.03196
- Title
- Neural Network Approach to Scaling Analysis of Critical Phenomena
- Author
- Ryosuke Yoneda and Kenji Harada
- Abstract
- Determining the universality class of a system exhibiting critical phenomena is one of the central problems in physics. As methods for determining this universality class from data, polynomial regression, which is less accurate, and Gaussian process regression, which provides high accuracy and flexibility but is computationally heavy, have been proposed. In this paper, we propose a method by a regression method using a neural network. The computational complexity is only linear in the number of data points. We demonstrate the proposed method for the finite-size scaling analysis of critical phenomena on the two-dimensional Ising model and bond percolation problem to confirm the performance. This method efficiently obtains the critical values with accuracy in both cases.
- Comments
- 10 pages, 9 figures
- Preprint
- arXiv:2209.01777
- Date
- Aug 26, 2022
- Conference (invited talk)
- The 15th Asia Pacific Physics Conference (APPC15), Korea (online)
- Title
- Tensor renormalization group study of the non-equilibrium critical fixed point of the one-dimensional contact process
- Abstract
- The steady-state of many stochastic systems is non-equilibrium. We studied the phase of non-equilibrium systems and the transition similar to equilibrium systems. In particular, the critical phase transition is interesting because we can define the non-equilibrium universality class. To confirm the existence of a non-equilibrium critical fixed point, we study the time evolution operator of one-dimensional contact processes by using a tensor renormalization group technique. The time evolution operators converge to universal critical tensors in the tensor renormalization group flow. The spectrums of critical tensors are strongly anisotropic but share the intrinsic structure each for the universality class. The integer structure for the universality class of compact-directed percolation in the time direction is consistent with the exact spectrum structure of the diffusion-annihilation process.
We will hold the oneline workshop, Tensor Network States: Algorithms and Applications (TNSAA) 2021-2022, from Jan. 17 to Jan. 21, 2022. The series of workshop, TNSAA, has been organized for the purposes of exchanging new developments, having discussions toward future studies, and providing intruductory talks for new generation of researchers about tensor networks.
- Date
- May 31, 2021
- Seminar
- StatPhys seminar at University of Tokyo, Hongo, Japan
- Title
- Universal spectrum structure on the nonequilibrium critical line of the one-dimensional Domany-Kinzel cellular automaton
- Abstract
-
The Domany-Kinzel(DK) cellular automaton is a stochastic time-evolutional system with an absorbing state from which the system cannot escape and a canonical model for nonequilibrium critical phenomena[1]. We introduce the tensor network method as a new tool to study it. Estimating the entropy of the DK automaton with a matrix product state representation of distribution, we reported a new cusp of the Renyi entropy in the active phase of the DK cellular automaton[2]. We recently applied a tensor renormalization group method to transfer matrices at the nonequilibrium critical point of the DK cellular automaton, confirming a universal spectrum structure[3]. In this talk, we will report our results with a brief review of models and methods.
[1] M. Henkel, H. Hinrichsen, and S. Lübeck, Non-Equilibrium Phase Transitions. Volume 1: Absorbing Phase Transitions, Vol. 1 (Springer, 2008).
[2] K. H. and N. Kawashima, Entropy Governed by the Absorbing State of Directed Percolation, Physical Review Letters 123, 090601 (2019).
[3] K. H., Universal spectrum structure at nonequilibrium critical points in the (1+1)-dimensional directed percolation, arXiv:2008.10807.
- Title
- Critical exponents in coupled phase-oscillator models on small-world networks
- Reference
- Physical Review E 102, 062212 (2020)
- DOI
- 10.1103/PhysRevE.102.062212
- Author
- Ryosuke Yoneda, Kenji Harada, and Yoshiyuki Y. Yamaguchi
- Abstract
- A coupled phase-oscillator model consists of phase oscillators, each of which has the natural frequency obeying a probability distribution and couples with other oscillators through a given periodic coupling function. This type of model is widely studied since it describes the synchronization transition, which emerges between the nonsynchronized state and partially synchronized states. The synchronization transition is characterized by several critical exponents, and we focus on the critical exponent defined by coupling strength dependence of the order parameter for revealing universality classes. In a typical interaction represented by the perfect graph, an infinite number of universality classes is yielded by dependency on the natural frequency distribution and the coupling function. Since the synchronization transition is also observed in a model on a small-world network, whose number of links is proportional to the number of oscillators, a natural question is whether the infinite number of universality classes remains in small-world networks irrespective of the order of links. Our numerical results suggest that the number of universality classes is reduced to one and the critical exponent is shared in the considered models having coupling functions up to second harmonics with unimodal and symmetric natural frequency distributions.
- Comments
- 8 pages, 8 figures
- Preprint
- arXiv:2007.04539
- Preprint
- arXiv:2008.10807
- Author
- Kenji Harada
- Abstract
- Using a tensor renormalization group method with oblique projectors for an anisotropic tensor network, we confirm that the rescaled spectrum of transfer matrices at nonequilibrium critical points in the (1+1)-dimensional directed percolation, a canonical model of nonequilibrium critical phenomena, is scale-invariant and its structure is universal.
- Comments
- 6 pages, 7 figures

- Preprint
- arXiv:2007.04539
- Title
- Critical exponents in coupled phase-oscillator models on small-world networks
- Author
- Ryosuke Yoneda, Kenji Harada, Yoshiyuki Y. Yamaguchi
- Abstract
- A coupled phase-oscillator model consists of phase-oscillators, each of which has the natural frequency obeying a probability distribution and couples with other oscillators through a given periodic coupling function. This type of models is widely studied since it describes the synchronization transition, which emerges between the non-synchronized state and partially synchronized states, and which is characterized by the critical exponents. Among them, we focus on the critical exponent defined by coupling strength dependence of the order parameter. The synchronization transition is not limited in the all-to-all interaction, whose number of links is of O(N2) with N oscillators, and occurs in small-world networks whose links are of O(N). In the all-to-all interaction, values of the critical exponent depend on the natural frequency distribution and the coupling function, classified into an infinite number of universality classes. A natural question is in small-world networks, whether the dependency remains irrespective of the order of links. To answer this question we numerically compute the critical exponent on small-world networks by using the finite-size scaling method with coupling functions up to the second harmonics and with unimodal and symmetric natural frequency distributions. Our numerical results suggest that, for the continuous transition, the considered models share the critical exponent 1/2, and that they are collapsed into one universality class.
- Comments
- 7 pages, 7 figures
- Title
- Finite-m scaling analysis of Berezinskii-Kosterlitz-Thouless phase transitions and entanglement spectrum for the six-state clock model
- Reference
- Physical Review E 101, 062111 (2020)
- DOI
- 10.1103/PhysRevE.101.062111
- Author
- Hiroshi Ueda, Kouichi Okunishi, Kenji Harada, Roman Krčmár, Andrej Gendiar, Seiji Yunoki, and Tomotoshi Nishino
- Abstract
- We investigate the Berezinskii-Kosterlitz-Thouless transitions for the square-lattice six-state clock model with the corner-transfer matrix renormalization group (CTMRG). Scaling analyzes for effective correlation length, magnetization, and entanglement entropy with respect to the cutoff dimension m at the fixed point of CTMRG provide transition temperatures consistent with a variety of recent numerical studies. We also reveal that the fixed point spectrum of the corner transfer matrix in the critical intermediate phase of the six-state clock model is characterized by the scaling dimension consistent with the c=1 boundary conformal field theory associated with the effective Z_6 dual sine-Gordon model.
- Comments
- 7 pages, 7 figures
- Preprint
- arXiv:2001.10176
- Preprint
- arXiv:2001.10176
- Author
- Hiroshi Ueda, Kouichi Okunishi, Kenji Harada, Roman Krčmár, Andrej Gendiar, Seiji Yunoki, and Tomotoshi Nishino
- Abstract
- We investigate the Berezinskii-Kosterlitz-Thouless transitions for the square-lattice six-state clock model with the corner-transfer matrix renormalization group (CTMRG). Scaling analyzes for effective correlation length, magnetization, and entanglement entropy with respect to the cutoff dimension m at the fixed point of CTMRG provide transition temperatures consistent with a variety of recent numerical studies. We also reveal that the fixed point spectrum of the corner transfer matrix in the critical intermediate phase of the six-state clock model is characterized by the scaling dimension consistent with the c=1 boundary conformal field theory associated with the effective Z_6 dual sine-Gordon model.
- Comments
- 7 pages, 7 figures
- Conference: Tensor Network States: Algorithms and Applications (TNSAA) 2019-2020
- Invited talk: "New numerical approaches for directed percolation"
- Date: Dec. 4, 2019
- Conference dates: Dec. 4-6, 2019
- Venue: NCCU, Taipei, TAIWAN
- URL:https://tnsaa7.github.io
We have uploaded videos of lectures and seminars onto YouTube as follows.
- TITLE
- Entropy Governed by the Absorbing State of Directed Percolation
- REFERENCE
- Physical Review Letters 123, 090601 (2019)
- DOI
- 10.1103/PhysRevLett.123.090601
- AUTHOR
- Kenji Harada and Naoki Kawashima
- ABSTRACT
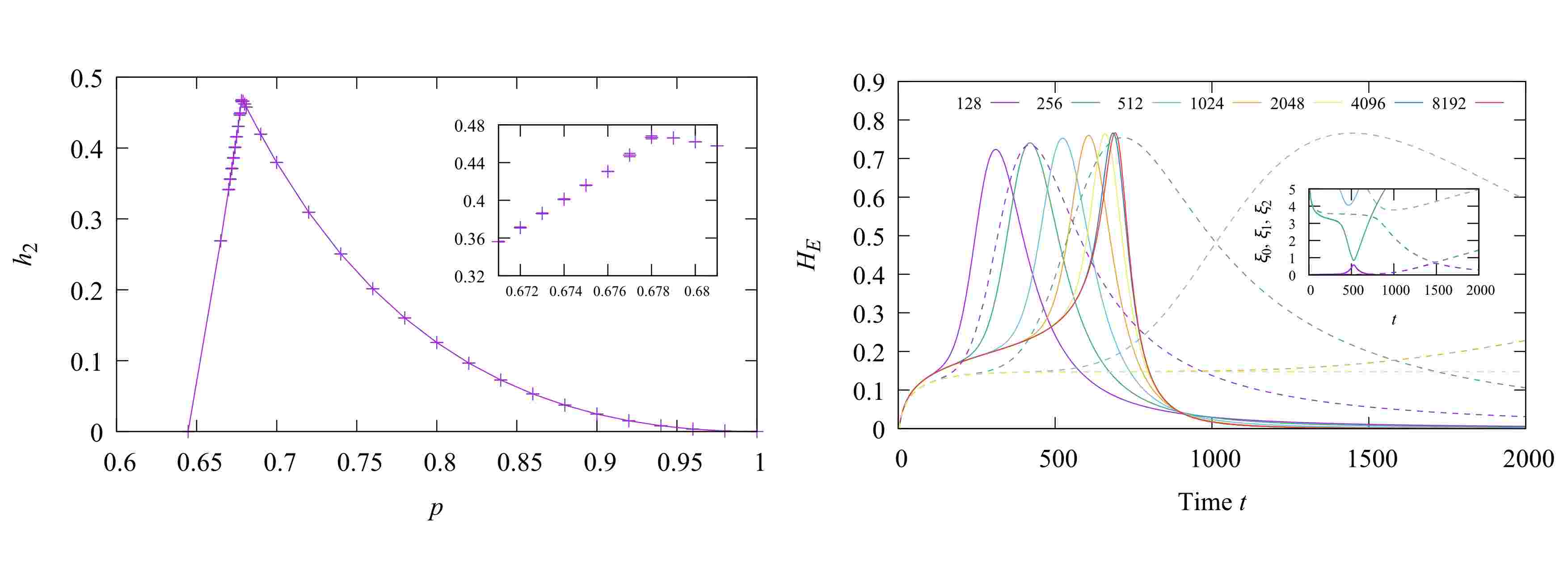
- We investigate the informational aspect of (1+1)-dimensional directed percolation, a canonical model of a nonequilibrium continuous transition to a phase dominated by a single special state called the “absorbing” state. Using a tensor network scheme, we numerically calculate the time evolution of state probability distribution of directed percolation. We find a universal relaxation of Rényi entropy at the absorbing phase transition point as well as a new singularity in the active phase, slightly but distinctly away from the absorbing transition point. At the new singular point, the second-order Rényi entropy has a clear cusp. There we also detect a singular behavior of “entanglement entropy,” defined by regarding the probability distribution as a wave function. The entanglement entropy vanishes below the singular point and stays finite above. We confirm that the absorbing state, though its occurrence is exponentially rare in the active phase, is responsible for these phenomena. This interpretation provides us with a unified understanding of time evolution of the Rényi entropy at the critical point as well as in the active phase.
- Title: Tensor network technique for stochastic process
- Date: July 17, 2019
- Title: Informational aspect of directed percolation problem
- Date: July 22, 2019
- Venue: The Institute for Solid State Physics, The University of Tokyo, JAPAN
- URL: http://www.issp.u-tokyo.ac.jp/public/caqmp2019/
- Preprint
- arXiv:1902.10479
- Author
- Kenji Harada and Naoki Kawashima
- Abstract
- We investigate the informational aspect of (1+1)-dimensional directed percolation(DP), a canonical model of a non-equilibrium continuous transition to a phase dominated by a single special state called the "absorbing" state. Using a tensor network scheme, we numerically calculate the time evolution of state probability distribution of DP. We find a universal relaxation of Renyi entropy at the absorbing phase transition point and a new singularity in the active phase where the second-order Renyi entropy has a cusp and the dynamical behavior of entanglement entropy changes from asymptotically-complete disentanglement to finite entanglement. We confirm that the absorbing state, though its occurrence is exponentially rare in the active phase, is responsible for these phenomena. This interpretation provides us with a unified understanding of time-evolution of the Renyi entropy at the critical point as well as in the active phase.
- Comments
- 6(=4+1.5) pages, 8(=5+3) figures
- Conference: Tensor Network States: Algorithms and Applications (TNSAA) 2018-2019
- Invited talk: "Entropy of the (1+1)-dimensional directed percolation"
- Conference dates: 3-6 December 2018
- Venue: R-CCS Kobe, JAPAN
- URL:http://quattro.phys.sci.kobe-u.ac.jp/kobe_2018/TNSAA2018-19.html
- Title: "Entropy of the (1+1)-dimensional directed percolation"
- Conference: International Conference on Advances in Physics of Emergent orders in Fluctuations (APEF2018)
- Conference dates: November 12-15, 2018
- Venue: The University of Tokyo, Tokyo, JAPAN
- URL:https://apef2018.org
REFERENCE Physical Review B 97 (2018) 045124
DOI 10.1103/PhysRevB.97.045124
AUTHOR Kenji Harada
ABSTRACT
We introduce an entanglement branching operator to split a composite entanglement flow in a tensor network which is a promising theoretical tool for many-body systems. We can optimize an entanglement branching operator by solving a minimization problem based on squeezing operators. The entanglement branching is a new useful operation to manipulate a tensor network. For example, finding a particular entanglement structure by an entanglement branching operator, we can improve a higher-order tensor renormalization group method to catch a proper renormalization flow in a tensor network space. This new method yields a new type of tensor network states. The second example is a many-body decomposition of a tensor by using an entanglement branching operator. We can use it for a perfect disentangling among tensors. Applying a many-body decomposition recursively, we conceptually derive projected entangled pair states from quantum states that satisfy the area law of entanglement entropy.
Wei-Lin is a student in a doctoral course of National Taiwan University. His stay is financially supported by Japan-Taiwan Exchange Association.
- International Symposium on Fluctuation and Structure out of Equilibrium 2017 (SFS2017)
-
- Date of poster presentation: 14:50 ~ 16:50, 20th Nov. 2017.
- Conference: International Symposium on Fluctuation and Structure out of Equilibrium 2017
- Conference dates: From 20th Nov. 2017 to 23th Nov. 2017.
- Venue: Sendai International Center, Sendai, Japan.
- URL:http://sfs-dynamics.jp/sfs2017/
- Title: "Entanglement branching operator" (invited)
-
- Date of presentation: 14:00 ~ 15:00, 6th Nov. 2017.
- Conference: Novel Quantum States in Condensed Matter 2017
- Conference dates: From 23th Oct. 2017 to 24th Nov. 2017.
- Venue: Yukawa Institute for Theoretical Physics, Kyoto University, Kyoto, Japan.
- URL:http://www2.yukawa.kyoto-u.ac.jp/~nqs2017.ws/index.php
- Preprint
- arXiv:1710.01830
- Abstract
- We introduce an entanglement branching operator to split a composite entanglement flow in a tensor network which is a promising theoretical tool for many-body systems. We can optimize an entanglement branching operator by solving a minimization problem based on squeezing operators. The entanglement branching is a new useful operation to manipulate a tensor network. For example, finding a particular entanglement structure by an entanglement branching operator, we can improve a higher-order tensor renormalization group method to catch a proper renormalization flow in a tensor network space. This new method yields a new type of tensor network states. The second example is a many-body decomposition of a tensor by using an entanglement branching operator. We can use it for a perfect disentangling among tensors. Applying a many-body decomposition recursively, we conceptually derive projected entangled pair states from quantum states that satisfy the area law of entanglement entropy.
- Preprint
- arXiv:1710.01830
- Author
- Kenji Harada
- Abstract
- We introduce an entanglement branching operator to split a composite entanglement flow in a tensor network which is a promising theoretical tool for many-body systems. We can optimize an entanglement branching operator by solving a minimization problem based on squeezing operators. The entanglement branching is a new useful operation to manipulate a tensor network. For example, finding a particular entanglement structure by an entanglement branching operator, we can improve a higher-order tensor renormalization group method to catch a proper renormalization flow in a tensor network space. This new method yields a new type of tensor network states. The second example is a many-body decomposition of a tensor by using an entanglement branching operator. We can use it for a perfect disentangling among tensors. Applying a many-body decomposition recursively, we conceptually derive projected entangled pair states from quantum states that satisfy the area law of entanglement entropy.
- Comments
- 9 pages, 11 figures
From 5 Feb. 2017 to 18 Feb. 2017, Workshop "Entanglement in Strongly Correlated Systems" , the Centro de Ciencias de Benasque Pedro Pascual, Benasque, Spain.
- Title: "General Entanglement Branching in a Tensor Network" (invited)
- Date of presentation: 14th Dec. 2016.
- Conference: Fourth Workshop on Tensor Network States: Algorithms and Applications
- Conference dates: From 12th Dec. 2016 to 15th Dec. 2016.
- Venue: National Center for Theoretical Sciences, Hsinchu, Taiwan
- URL:http://www.phys.cts.nthu.edu.tw/actnews/index.php?Sn=318
- Title: "Branching and tensor network" (invited)
- Date: June 27, 2016
- Venue: The Institute for Solid State Physics, The University of Tokyo, JAPAN
- URL: http://www.issp.u-tokyo.ac.jp/public/tnqmp2016/
REFERENCE Physical Review B 92 (2015) 134404
DOI 10.1103/PhysRevB.92.134404
AUTHOR Tsuyoshi Okubo, Kenji Harada, Jie Lou, and Naoki Kaishima
ABSTRACT
The SU(N) symmetric antiferromagnetic Heisenberg model with multicolumn representations on the two- dimensional square lattice is investigated by quantum Monte Carlo simulations. For the representation of a Young diagram with two columns, we confirm that a valence-bond solid (VBS) order appears as soon as the Néel order disappears at N = 10, indicating no intermediate phase. In the case of the representation with three columns, there is no evidence for either the Néel or the VBS ordering for N >= 15. This is actually consistent with the large-N theory, which predicts that the VBS state immediately follows the Néel state, because the expected spontaneous order is too weak to be detected.
REFERENCE Physical Review E 92 (2015) 012106
DOI 10.1103/PhysRevE.92.012106
AUTHOR Kenji Harada
ABSTRACT
Scaling analysis, in which one infers scaling exponents and a scaling function in a scaling law from given data, is a powerful tool for determining universal properties of critical phenomena in many fields of science. However, there are corrections to scaling in many cases, and then the inference problem becomes ill-posed by an uncontrollable irrelevant scaling variable. We propose a new kernel method based on Gaussian process regression to fix this problem generally. We test the performance of the new kernel method for some example cases. In all cases, when the precision of the example data increases, inference results of the new kernel method correctly converge. Because there is no limitation in the new kernel method for the scaling function even with corrections to scaling, unlike in the conventional method, the new kernel method can be widely applied to real data in critical phenomena.
NOTE
The reference code of this new method is prepared at http://kenjiharada.github.io/BSA/
BSA toolkit is a reference code of a new method for scaling analysis of critical phenomena. Using Bayesian inference, we automatically estimate critical point and indices. We fixed a bug for the output of scaling function with the option "-f 1". This bug does not affect the inference result of parameters.
PREPRINT arXiv:1504.05332
AUTHOR Tsuyoshi Okubo, Kenji Harada, Jie Lou, Naoki Kaishima
ABSTRACT
The SU(N) symmetric antiferromagnetic Heisenberg model with
multi-column representations on the two-dimensional square lattice is
investigated by quantum Monte Carlo simulations. For the representation of
Young diagram with two columns, we confirm that a valence-bond solid order
appears as soon as the N'eel order disappears at N = 10 indicating no
intermediate phase. In the case of the representation with three columns, there
is no evidence for both of the N'eel and the valence-bond solid ordering for
N >= 15. This is actually consistent with the large-N theory, which
predicts that the VBS state immediately follows the N'eel state, because the
expected spontaneous order is too weak to be detected.
REFERENCE Physical Review B 91 (2015) 094414
DOI 10.1103/PhysRevB.91.094414
AUTHOR Takafumi Suzuki, Kenji Harada, Haruhiko Matsuo, Synge Todo, and Naoki Kaishima
ABSTRACT
We investigate thermal phase transitions to a valence-bond solid phase in SU(N) Heisenberg models with four- or six-body interactions on a square or honeycomb lattice, respectively. In both cases, a thermal phase transition occurs that is accompanied by rotational symmetry breaking of the lattice. We perform quantum Monte Carlo calculations in order to clarify the critical properties of the models. The estimated critical exponents indicate that the universality classes of the square- and honeycomb-lattice cases are identical to those of the classical XY model with a Z4 symmetry-breaking field and the three-state Potts model, respectively. In the square-lattice case, the thermal exponent, ν, monotonically increases as the system approaches the quantum critical point, while the values of the critical exponents, η and γ/ν, remain constant. From a finite-size scaling analysis, we find that the system exhibits weak universality, because the Z4 symmetry-breaking field is always marginal. In contrast, ν in the honeycomb-lattice case exhibits a constant value, even in the vicinity of the quantum critical point, because the Z3 field remains relevant in the SU(3) and SU(4) cases.
Title: ''Quantum Monte Carlo study of Quantum Criticality on SO(N) Bilinear-biquadratic Chains'' Date: Feb. 18, 2015 Conference: From Feb. 18, 2015 to Feb. 21, 2015, International Workshop on New Frontier of Numerical Methods for Many-Body Correlations ― Methodologies and Algorithms for Fermion Many-Body Problems , Hongo Campus, The University of Tokyo, Japan.
Title: ''Quantum Monte Carlo study of Quantum Criticality on SO(N) Bilinear Biquadratic Chains'' Date: Jan. 9, 2015 Conference: From Jan. 7, 2015 to Jan. 11, 2015, the 9th International Conference on Computational Physics (ICCP9) , National University of Singapore, Singapore.
Conference:
10sor network workshop --- Field 2x5 joint workshop on new algorithms for quantum manybody problems ---
Date: November 25, 2014, Tuedsay
Venue: Kashiwa Future Center, Kashiwa, Chiba, Japan
Title: "MERA tensor network and its application on quantum frustrated magnets"
From Nov. 4, 2014 to Dec. 2, 2014, the YITP long-term Workshop "Novel Quantum States in Condensed Matter 2014" (NQS2014) , YITP, Kyoto university, Kyoto, Japan.
From 20 Oct. 2014 to 22 Oct. 2014, the CMSI International Workshop 2014: Tensor Network Algorithms in Materials Science , RIKEN Advanced Institute for Computational Science 6F auditorium 7-1-26, Port Island South, Kobe, 650-0047, Japan.
The reference code of the new kernel method is added in the toolkit BSA. The new code can easily do the finite-size scaling analysis with or without corrections to scaling.
PREPRINT arXiv:1410.3622
AUTHOR Kenji Harada
ABSTRACT
Scaling analysis, in which one infers scaling exponents and a scaling function in a scaling law from given data, is a powerful tool for determining universal properties of critical phenomena in many fields of science. However, there are corrections to scaling in many cases, and then the inference problem becomes ill-posed by an uncontrollable irrelevant scaling variable. We propose a new kernel method based on Gaussian process regression to fix this problem generally. We test the performance of the new kernel method for some example cases. In all cases, when the precision of the example data increases, inference results of the new kernel method correctly converge. Because there is no limitation in the new kernel method for the scaling function even with corrections to scaling, unlike in the conventional method, the new kernel method can be widely applied to real data in critical phenomena.
REFERENCE JPS Conf. Proc. 3 , 014031 (2014) [7 pages]
Proceedings of the International Conference on Strongly Correlated Electron Systems (SCES2013)
DOI 10.7566/JPSCP.3.014031
AUTHORKenji Harada
ABSTRACT
We will introduce tensor network states in the variational calculation of ground states for quantum frustrated magnets. In particular, we will report the performance of MERA tensor network for an S = 1/2 antiferromagnetic Heisenberg model on a spatially anisotropic triangular lattice, which is an effective model of Mott insulators on a triangular layer of organic charge transfer salts.
REFERENCE Physical Review B 89 (2014) 134422
DOI 10.1103/PhysRevB.89.134422
PREPRINT arXiv:1312.2643
AUTHOR Kouichi Okunishi and Kenji Harada
ABSTRACT
Using a generalized Jordan-Wigner transformation combined with the defining representation of the SO(N) spin, we map the SO(N) bilinear-biquadratic(BLBQ) spin chain into the N-color bosonic particle model. We find that, when the Jordan-Wigner transformation disentangles the symmetry-protected topological entanglement, this bosonic model becomes negative-sign free in the context of quantum Monte-Carlo simulation. For the SO(3) case, moreover, Kennedy-Tasaki's transformation for the S=1 BLBQ chain, which is also a topological disentangler, derives the same bosonic model through the dimer-R bases. We present temperature dependence of energy, entropy and string order parameter for the SO(N=3, 4, 5) BLBQ chains by the world-line Monte-Carlo simulation for the N-color bosonic particle model.
REFERENCE Physical Review Letters 112 (2014) 140603
DOI 10.1103/PhysRevLett.112.140603
PREPRINT arXiv:1307.0328
AUTHOR Akiko Masaki-Kato, Takafumi Suzuki, Kenji Harada, Synge Todo, Naoki Kaishima
ABSTRACT
Based on the worm algorithm in the path-integral representation, we propose a general quantum Monte Carlo algorithm suitable for parallelizing on a distributed-memory computer by domain decomposition. Of particular importance is its application to large lattice systems of bosons and spins. A large number of worms are introduced and its population is controlled by a fictitious transverse field. For a benchmark, we study the size-dependence of the Bose-condensation order parameter of the hardcore Bose-Hubbard model with $L\times L\times \beta t = 10240\times 10240\times 16$, using 3200 computing cores, which shows good parallelization efficiency.
From 3 March 2014 to 7 March 2014,
- Talk (Collaborator) "Parallelized Multi-Worm Algorithm for Large Scale Quantum Monte-Carlo simulations" C1.00284
- Talk (Collaborator) "Thermal phase transitions to valence-bond-solid states in the two dimensional SU(N) Heisenberg models" F7.00010
- Talk (Representative) "Possibility of Deconfined Criticality in SU(N) Heisenberg Models at Small N" S27.00013
APS March Meeting 2014, Denver, Colorado, USA.
Toolkit BSA is a reference code of a new method for scaling analysis of critical phenomena. Using Bayesian inference, we automatically estimate critical point and indices. This toolkit is announced in the MateriApps site.
REFERENCE Physical Review B 88 (2013) 220408(R)
DOI 10.1103/PhysRevB.88.220408
PREPRINT arXiv:1307.0501v2
AUTHOR
Kenji Harada, Takafumi Suzuki, Tsuyoshi Okubo, Haruhiko Matsuo, Jie Lou, Hiroshi Watanabe, Synge Todo, and Naoki Kaishima
ABSTRACT
To examine the validity of the scenario of the deconfined critical phenomena, we carry out a quantum Monte Carlo simulation for the SU($N$) generalization of the Heisenberg model with four-body and six-body interactions. The quantum phase transition between the SU($N$) N\'eel and valence-bond solid phases is characterized for $N=2,3,$ and $4$ on the square and honeycomb lattices. While finite-size scaling analysis works well up to the maximum lattice size ($L=256$) and indicates the continuous nature of the phase transition, a clear systematic change towards the first-order transition is observed in the estimates of the critical exponent $y \equiv 1/\nu$ as the system size increases. We also confirm the relevance of a squared valence-bond solid field $\Psi^2$ for the SU(3) model.
PREPRINT arXiv:1312.2643
AUTHOR Kouichi Okunishi and Kenji Harada
ABSTRACT
Using a generalized Jordan-Wigner transformation combined with the defining representation of the SO(N) spin, we map the SO(N) bilinear-biquadratic(BLBQ) spin chain into the N-color bosonic particle model. We find that, when the Jordan-Wigner transformation disentangles the symmetry-protected topological entanglement, this bosonic model becomes negative-sign free in the context of quantum Monte-Carlo simulation. For the SO(3) case, moreover, Kennedy-Tasaki's transformation for the S=1 BLBQ chain, which is also a topological disentangler, derives the same bosonic model through the dimer-R bases. We present temperature dependence of energy, entropy and string order parameter for the SO(N=3, 4, 5) BLBQ chains by the world-line Monte-Carlo simulation for the N-color bosonic particle model.
From 2 Dec. 2013 to 5 Dec. 2013, Taipei Tensor Network Workshop 2013 , National Taiwan University, Taipei, Taiwan.
From 21 Oct. 2013 to 22 Oct. 2013,
CMSI International Symposium 2013:
"Extending the power of computational materials sciences with K-computer"
,
Ito International Research Center in the University of Tokyo, Hongo Campus in Tokyo, Japan.
From 16 Oct. 2013 to 18 Oct. 2013, Satellite Meeting 2013 in Kobe: "CMSI Kobe International Workshop 2013: Recent Progress in Tensor Network Algorithms" , RIKEN Advanced Institute for Computational Science, Kobe, Japan.
From 28 July 2013 to 31 July 2013, "Statistical Physics of Quantum Matter", National Taiwan University, Taipei, Taiwan.
PREPRINT arXiv:1307.0328
AUTHOR Akiko Masaki-Kato, Takafumi Suzuki, Kenji Harada, Synge Todo, Naoki Kaishima
ABSTRACT
Based on the worm algorithm in the path-integral representation, we propose a general quantum Monte Carlo algorithm suitable for parallelizing on a distributed-memory computer by domain decomposition. Of particular importance is its application to large lattice systems of bosons and spins. A large number of worms are introduced and its population is controlled by a fictitious transverse field. For a benchmark, we study the size-dependence of the Bose-condensation order parameter of the hardcore Bose-Hubbard model with $L \times L \times \beta t =10240 \times 10240 \times 16$, using 3200 computing cores, which shows good parallelization efficiency.
From 18 March 2013 to 22 March 2013, APS March Meeting 2013, Baltimore, Maryland, USA.
PREPRINT arXiv:1212.1999
AUTHOR Jie Lou, Takafumi Suzuki, Kenji Harada, and Naoki Kaishima
ABSTRACT
We performed variational calculation based on the multi-scale entanglemnt renormalization ansatz, for the antiferromagnetic Heisenberg model on a Shastry Sutherland lattice (SSL). Our results show that at coupling ratio J'/J= 0.687(3), the system undergoes a quantum phase transition from the orthogonal dimer order to the plaquette valence bond solid phase, which then transits into the antiferromagnetic order above J'/J=0.75. In the presence of an external magnetic field, our calculations show clear evidences of various magnetic plateaux in systems with different coupling ratios range from 0.5 to 0.69. Our calculations are not limited to the small coupling ratio region, and we are able to show strong evidence of the presence of several supersolid phases, including ones above 1/2 and 1/3 plateaux. Such supersolid phases, which feature the coexistence of compressible superfluidity and crystalline long range order in triplet excitations, emerge at relatively large coupling ratio (J'/J>0.5). A schematic phase diagram of the SSL model in the presence of magnetic field is provided.
REFERENCE Physical Review B 86 (2012) 184421
DOI 10.1103/PhysRevB.86.184421
PREPRINT arXiv:1208.4306
AUTHOR
Kenji Harada
ABSTRACT
The ground state of an $S=1/2$ antiferromagnetic Heisenberg model on a spatially anisotropic triangular lattice, which is an effective model of Mott insulators on a triangular layer of organic charge transfer salts or Cs2CuCl4, is numerically studied. We apply a numerical variational method by using a tensor network with entanglement renormalization, which improves the capability of describing a quantum state. Magnetic ground states are identified for 0.7 <= J2 / J1 <= 1 in the thermodynamic limit, where J1 and J2 denote the innerchain and interchain coupling constants, respectively. Except for the isotropic case (J1 = J2), the magnetic structure is spiral with an incommensurate wave vector that is different from the classical one. The quantum fluctuation weakens the effective coupling between chains, but the magnetic order remains in the thermodynamic limit. In addition, the incommensurate wave number is in good agreement with that of the series expansion method.